EE 420L Engineering Electronics II Lab

Lab 5 - Op–amps III, the op–amp integrator


Francisco Mata Carlos

 email: matacarl@unlv.nevada.edu

 3/13/19

 

Pre-lab:

 

 

Lab description:

The goal of this lab is to calculate the unity-gain frequency of the figure below, and to design and simulate a triangle wave from a square wave input.

 

This lab will utilize the LM324 op-amp (LM324.pdf), and for questions and experiments, VCC+ = +5V and VCC- = 0V

Experiment 1:

a)    Calculate the frequency response of the following circuit. Ensure you show your clear hand calculations.

o    What can you neglect to simplify the calculation?

o    Does the circuit work if you remove the 100k? Why or why not?

o    Does the 100k have much of an effect on the frequency response?

b)    Verify your calculations with experimental results.

c)    Show, at the unity-gain frequency of the integrator, that the input and the output have the same peak values.

o    Is the phase shift between the input and the output what you expect? Why or why not?

 

 

 

a)       Calculate the frequency response of the following circuit. Ensure you show your clear hand calculations.

 

1)  What can you neglect to simplify the calculation?

First, we can neglect the DC circuit and the Common node voltage and focus only on AC. Second, R2 (100K) is not affecting much

The frequency response, since we can divide by R2 and have the same results.

 

2)  Does the circuit work if you remove the 100k? Why or why not?

 

Circuit without 100k resistor

frequency

LTspice results

Experimental results

 

 

 

 

 

10Hz

 

 

 

159Hz

 

 

1kHz

 

 

10kHz

 

Over 800kHz

 

               The output rails

 

 

Circuit with 100k resistor

frequency

LTspice results

Experimental results

 

 

 

 

 

10Hz

 

 

 

 

159Hz

 

 

 

1kHz

 

 

 

 

10kHz

 

 

Over 600KHz

 

The output rails

 

Note: The results shown above for the same circuit with 100k and without, demonstrate that the 100K resistor does not

        affect much the frequency response

 

 

3)  Does the 100k have much of an effect on the frequency response?

The 100k resistor does not have much effect on the frequency response because as shown in the hand calculations section

the top and bottom of the magnitude is being multiplied by R2, which causes to have the same ratio as if it wasn’t there.

 

B) Verify your calculations with experimental results

Show, at the unity-gain frequency of the integrator, that the input and the output have the same peak values.

Is the phase shift between the input and the output what you expect? Why or why not?


  
     

 

       

   

 

Experiment 2:

a)   Next, design, simulate, and build a square-wave to triangle wave generation circuit.

b)   Assume the input/output frequency is 10 kHz and the output ramp must swing from 1 to 4 V centered around 2.5 V.

c)   Show all calculations and discuss the trade-offs (capacitor and resistor values, input peak, min, and average, etc.)

 

a)            Next, design, simulate, and build a square-wave to triangle wave generation circuit

 

            

 

 

b)            Assume the input/output frequency is 10 kHz and the output ramp must swing from 1 to 4 V centered around 2.5 V.

 

    LTspice Simulations

       

 

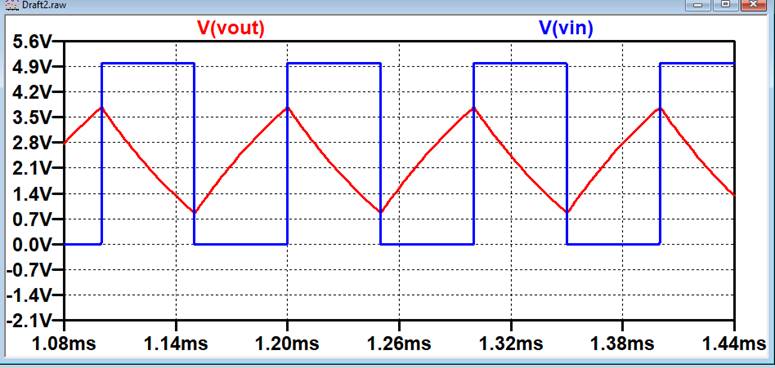
      Experimental Results

             

    

c)       Show all calculations and discuss the trade-offs (capacitor and resistor values, input peak, min, and average, etc.)

         The calculations for this circuit are shown above in part a).

         

          Trades-offs

         

          For experiment 1, the trade-ff is the values of the resistors in relation to the capacitor to crate the unity gain frequency. By increasing the

          unity frequency gain, the value of R1 decreases, which also increases the total gain of the output, if R2 is constant. Thus, this increase in frequency

          increases the input offset voltage, which increases the offset of the output.


 

 

          For experiment 2, the trade-ff is very similar as for experiment 1. We want R2 and R1 to have a small gain because we don’t want the input

          offset voltage to affect the swing of the output. But if R1 is increased in value, then the capacitor value will have to be decreased in order to

          maintain the ramp that is desired.

       

 

           Return to student lab reports


           Return to labs